Does the Special Theory of Relativity support relationalism about space-time? — UCL’s Philosophy of Space & Time (2019)

Tiny planet photo effect of me in New York

1. Introduction

There is an ongoing debate among philosophers and physicists as to what the Special Theory of Relativity (STR) states as a theory and what kind of nature of space, time, or spacetime should be espoused assuming STR’s veracity — relationalist or substantivalist. I will submit two arguments. First, STR supports relationism in a narrow way. This is because Einstein aimed to eliminate absolute quantities of motion from physics, thus formulating a theory that satisfies at least one kind of ‘strict’ relationism. But not the kind of relationism advocated by Leibniz. Second, I contend that in order to account for observable phenomena, STR is absolutist about the geometrical structure of spacetime and introduces new ‘absolute’ ontological items like space-time intervals which may be treated as surrogates for Newton’s absolute time and space. In this sense, I will argue that STR is in a strong sense partially substantivalist.

I favour the interpretation of STR that requires us to posit an independently existing ‘manifold’ of spacetime points that exists over and above the material bodies and the relations between them (Teller, 1991, p363). This is because it provides a simpler and more perspicuous explanation than its rivals since the alternative would mean imbuing spacetime with less structure which would reduce our explanatory power and predictive success.

2. Exposition

Relationism vs absolutism

Relationism is the view that there exist just material bodies, standing in various spatial relationships. Relationists contend that there is no additional entity that exists independently of the bodies it comprises (Dasgupta, 2015, p601) and it is not possible that space could have existed devoid of all objects. The different types of relations include: part/whole, betweenness and congruence relations (Maudlin, 1993, p198). Absolutism is the opposing view that space ‘can be said to exist and to have specified features independently of the existence of ordinary material objects’, (Sklar, 1974, p161). That is, space has a reality that supervenes the objects it contains. Having an independent reality is normally taken to be the essential criterion for regarding something like a ‘substance’, hence absolutists are sometimes referred to as ‘substantivalists’ about space.

Historically, the debate between relationists and absolutists is couched in Leibnizian and Newtonian terms. However, I will show that the modern debate between relational and absolute theories of space becomes ambiguous in the context of special relativity and why we should be careful not to reach any hard conclusions about which metaphysical picture STR posits.

Gottfried Wilhelm Leibniz

Leibniz

Gottfried Wilhelm Leibniz (1646–1716), in his correspondence with Clarke in 1715–16, maintained the following version of a relationism:

1. ‘Space is not an entity in its own right, but merely a set of ways in which simultaneously existing bodies could be ordered.
2. All motion is relative to some body.
3. There is absolute motion of a body B, but it is not motion with respect to absolute space, but relative motion whose cause is in B.’ (Gardner, 1977, p219)

As Gardener (1977) explains, Leibniz’s supposition that space is an ‘order of coexistences’ indicates that the relations between bodies are solely relations of order and not of quantity (p219). Or, in Maudlin’s terms, the relations are topological (preserved under one-to-one bicontinuous deformations) rather than metric (preserved under rigid transformations) (2012, p6). Consequently, it may appear that Leibniz revokes Newton’s thesis that distance is a genuine relation between bodies.

From this perspective, Leibniz was a relationist in the further sense that he maintained that ‘distance is a real-valued three-place function of pairs of bodies and congruence standards, whose values are defined by a measuring procedure involving the standard’ (Gardner, 1977, p219).

Sir Isaac Newton

Newton

In his Principia, originally published in 1687, Isaac Newton (1643–1727) maintained the following version of absolute (or substantival) theory of space:

1. ‘Absolute space exists as an entity in its own right, not merely as a system of relations of bodies.
2. There is absolute motion.
3. Absolute motion is motion with respect to absolute space.
4. Distance is a two-place real-valued function of bodies or points, and is not to be identified by definition with the results of any particular sort of measuring process.’ (p216)

(1)-(3) are uttered explicitly in Newton (1999) where he says, ‘Absolute space, in its own nature, without relation to anything external, remains always similar and immovable.’ Moreover, ‘[absolute] place is a part of [absolute] space which a body takes up’, and ‘Absolute motion is the translation of a body from one absolute place into another’ (pp 6–7).

Further, Newton notes that there are coordinate systems of absolute space which have a body at some fixed coordinates, which he terms ‘relative spaces’. Relative motion can, therefore, be defined as ‘change of coordinates in a relative space, or as motion with respect to some body’ (Gardener, 1977, p216). (4) is more difficult to trace. Newton does say (pii) ‘Nor do those less defile the purity of mathematical and philosophical truths, who confound real quantities with their relations and sensible measures’, which may suggest (4). However, as Gardner (1977) explains, ‘the context concerns the distinction between absolute and relative motions and this quotation may refer to that distinction, rather than to that between a quantity (whether absolute or relative) and the result of a measurement’ (p217). But since Newton explicitly made a conceptual distinction between duration in absolute time and the number of cycles of any physical process, it is likely, albeit not definite, that he espoused the ‘analogous spatial thesis’ (i.e (4)) (pp 6–8).

Special Theory of Relativity (STR)

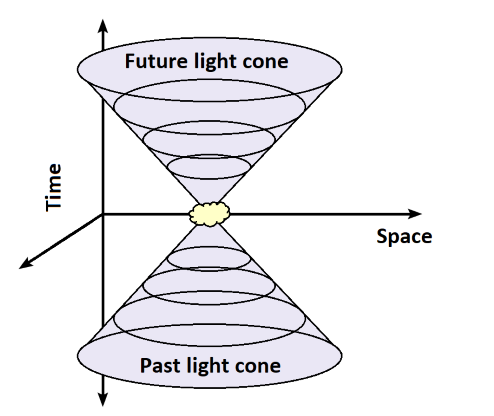
STR submits two principles (or postulates): (1) the equivalence of all inertial frames and (2) the constancy of the speed of light (Maudlin, 2012, p67). Newton’s view was that there exists an absolute, non-relative, spatial and temporal separation between any two events (Spring, 2002, p17.1). Conversely, the first and second principles of STR posit that such spatial and temporal separations can only be defined relative to an inertial (i.e. ‘non-accelerating’) frame of reference independently of the velocity of the light’s source. Moreover, these separations will differ depending on which inertial reference frame and spatial position are selected (Maudlin, 2012, p68). Rather than an invariant (i.e. ‘identical’) time interval between two events, there is an invariant spacetime interval (p70). Rather than investigating all facets of STR, I will focus on what sort of spacetime structure — relationist or substantivalist — STR postulates from these general principles.

Central to STR is an empirically verifiable fact about the behaviour of light in a vacuum. The second principle holds that the spacetime trajectory (or ‘worldline’) of light in a vacuum is independent of the relative or absolute state of motion or acceleration of the source or of the observer. That is, ‘the geometry of space-time alone determines the trajectory of light rays’ (Maudlin, 2012, p68). On this account, the set of space-time points a light signal will reach depends only upon the point, p, in spacetime from which the signal is sent.

Each spacetime point can be described as an ‘event’. An event is essentially a place-in-space-at-a-time. For example, the explosion of a firecracker occurs at a space-time point, which occurs only once and, ceteris paribus, has no spatial extension and takes up no time (p60). Events are the fundamental ingredients of the spatiotemporal ontology of Galilean spacetime. However, STR made the conceptual advance of replacing Galilean transformations of Newtonian mechanics, which treat length and temporal separation between two events as independent invariants (i.e. values of which do not change when observed from different reference frames) with the Lorentz transformations (i.e. the equations that relate measurements made in different reference frames) (p67). Accordingly, time and space cannot be defined independently from one another. Instead, space and time are interwoven into a single continuum known as “spacetime”. Whereas Galilean spacetime can be partitioned into sets of events that all happen simultaneously (p61), STR is restricted to the flat spacetime known as Minkowski space (p69). The aim of this essay is to investigate the physical structure of these events, that is, the geometry of space-time.

An event has a ‘past light-cone’ and a ‘future light-cone’. The future light-cone of p is defined as the set of spacetime points where light emitted from p (in a vacuum) might arrive. The past light-cone of p refers to one of a particular set of spacetime trajectories that a light signal traversed before reaching p (p68). From these empirical facts about light’s behaviour, we can derive two laws. First, the Law of Light states that ‘the trajectory of a light ray emitted from an event (in a vacuum) is a straight line on the future light-cone of that event’ (p73). Second, the Relativistic Law of Inertia states that ‘the trajectory of any physical entity subject to no external influences is a straight line in Minkowski space-time’ (p75).

3. My Critique

Relationism vindicated?

My first argument is that STR supports relationism in a narrow way. This is because Einstein aimed to eliminate absolute quantities of motion (i.e. absolute velocity and absolute rest) from physics, therefore, realising a theory of spacetime that retrospectively satisfies at least one kind of ‘strict’ relationism (Huggett and Hoefer, 2018). Evidence for this comes from three observations. First, unlike in Newtonian mechanics, in the Minkowski spacetime of STR absolute acceleration is treated as a curved trajectory with respect to space-time itself rather than as a derivative of absolute (constant) velocity, which is itself a derivative of absolute position (or ‘rest’) (Maudlin, 2012, p50). Constant velocity is to traverse equal amounts of space (in the same direction) in equal amounts of time. But this presupposes regions of space that remain constant over time and that have an inertial and a metrical structure (p62). To remain at absolute rest is to occupy the same set of points in space. By decoupling absolute acceleration from absolute velocity and absolute rest, STR seems to favour the relationist view that there are no genuine points of space that have a numerical identity over time which can be treated as a privileged reference frame. But only other bodies that a body, B, could move with respect to (Gardner, 1977, p219).

Further support for this claim can be found in Langevin’s Twins Paradox. The Twins phenomenon can be explained without having to attribute ‘rest’ to either twin: it is purely a question of space-time geometry (Maudlin, 2012, p83). Minkowski space-time, like Galilean space-time (and unlike Newtonian absolute space and time) supports no notion of rest (ibid). Provided we can identify the straight lines in the space-time diagram, and quantify the curvature of the second twin’s worldline using a revised equation of motion that calculates curvature, Maudlin grants that we can determine what Newton’s Laws predict in any given instance (p60). That is, we do not need to know the trajectories of individual points of absolute space.

Second, one might interpret interval measures in the Minkowski spacetime as being relational since the function that defines the Interval between events is not always positive-definite (p71). That is, the function can take the value zero for some pairs of distinct points. On this account, the Interval cannot be considered a distance: any pair of distinct points should have some nonzero distance between them (p63). This would support Leibniz’s view that distance is not a relation of two bodies (that have a fixed position in absolute space), but a relation which they bear to a third object called a congruence relation (Gardner, 1987, p216). Further support for this claim comes from the phenomenon of length contraction. As objects move through spacetime, space and time experience changes in measurement. The length of the objects ‘contract’ when they move by an observer at relativistic velocities (that is, those at a significant fraction of the speed of light) (Dalarsson and Dalarsson, 2015, p160). This is because in STR both spatial distances and time intervals are observer-relative (observers in different states of motion disagree about their sizes). The first principle of STR implies that the only way to measure the differences in these velocities (i.e. their spatial relation) is to relinquish a notion of a definite distance that can be measured by a privileged reference frame.

Further support for this claim comes from the Clock Hypothesis. The Clock Hypothesis states that ‘the amount of time that an accurate clock shows to have elapsed between two events is proportional to the Interval along the clock’s trajectory between those events, or, in short, clocks measure the Interval along their trajectories’ (Maudlin, 2012, p76). Since there is no absolute time or rest in STR, clocks cannot measure it. Instead, clocks that traverse different trajectories record different elapsed times between the same pair of events (ibid).

Superficially, STR seems to vindicate relatonism. However, I submit that the most we can infer from the previous argument is that in Newtonian physics there are features of the world that are ‘absolute’ which are ‘relative’ (rather than purely relational in the Leibniz sense) in STR. But not the kind of relationism advocated by Leibniz.

Substantivalism triumphs, mutatis mutandis

My second argument is that STR supports substantivalism in a strong sense. I submit this for three reasons. First, STR posits an objective geometrical structure to space-time that defines inertial trajectories for all bodies, regardless of the existence of other bodies (Maudlin, 2012, p80). Support for this claim comes from the Limiting Role of the Light-cone, which states that ‘the trajectory of any physical entity that goes through an event never goes outside the light-cone of that event’ (p75). That is, the temporal order of timelike events (i.e. events within the backwards or forwards light-cone of O) is absolute.

As Brown (2018) explains:

‘the unified spacetime manifold with the lightcone structure of Minkowski spacetime is more rigid than a pure Cartesian product of a three-dimensional spatial manifold and an independent one-dimensional temporal manifold. In contrast, the lightcone structure of Minkowski spacetime restricts the future of the point P0 to points inside the future null cone, i.e., P0 ± cdt, and as dt goes to zero, this range goes to zero, imposing a well-defined unique connection from each “infinitesimal” instant to the next, which of course is what the unification of space and time into a single continuum accomplishes.’

In other words, while the trajectory of an object is not defined by a privileged inertial frame (absolute space or aether), the geometry of spacetime itself is assumed to be absolute because it is assumed that spacetime has a metric so that we can talk about the amount of time that has elapsed between two events. The curved trajectory of an accelerating object is thus with respect to spacetime itself and so the notion of acceleration is genuinely absolute, and not defined in terms of an object’s relations to other objects.

Further evidence of this can be found in Maudlin’s rendition of the Twins Paradox and Newton’s Twin Globes experiment. In Maudlin’s version of the Twins Paradox, we have two twins, Twin A and Twin B. Initially, both twins are travelling inertially in spaceships along the same worldline and are carrying identical clocks. Twin A accelerates away from Twin B near the speed of light, then accelerates back to Twin B and returns to relative rest with respect to Twin B. The paradox is that when Twin A returns to Twin B she discovers that Twin B’s clock displays a later time than Twin A and has, therefore, aged more (Maudlin, 2012, p77). The relation between the twins is perfectly symmetrical (by virtue of Minkowski spacetime), that is, the spatial distance between them begins at zero, increases to some maximum value, and then decreases back to zero (Brown, 2018; Maudlin, 2012, p117). The distinction between the twins cannot, therefore, be explained in respect of their mutual spatial relations, but only in respect of how each of their individual worldlines is entrenched in the absolute metrical manifold of Minkowski spacetime.

On this account, the 4-dimensional spatio-temporal framework of Minkowski spacetime unsettles the claim that interval measures in the Minkowski spacetime are purely relational. This is because Minkowski spacetime implies that ‘proper length’ and ‘proper time’ give a measure of the length of a path connecting two events in timelike separation that is independent of the coordinates of the bodies in question and ‘correspond to the (frame-invariant) space-time interval between certain well-defined spacetime points’ (Huggett and Hoefer, 2018; Arthur, 2010, p4). In the Twin Globes experiment, as Maudlin (2012) explains, Newton’s explanation of the rotating globes is vindicated by STR given that there is an ’absolute fact about whether the twin globes are rotating about their common center of gravity or not’ (pp 23, 80). The tension in the rope connecting the globes cannot be explained in respect of their spatial relations, but only in respect of the (immovable) geometrical structure of spacetime (p80; Brown, 2018). Even in the interval-relational interpretation of STR, there are well-defined absolute accelerations and rotations (Huggett and Hoefer, 2018).

The second way STR undermines relationism is that there has traditionally been a strong association between relationism and the (implicit) notion of absolute simultaneity. This is because the ‘relations’ among events happening at the same time in a given reference frame were regarded as purely spatial, and it was necessary to posit a unique instantaneous point of time in which to evaluate those spatial relations (Brown, 2018). However, STR contends that simultaneity is not an absolute relation between events. This is because Minkowski space-time denies a preferred foliation of spacetime or privileged plain of simultaneity (Maudlin, 2012, p76, Pooley, 2001, p2). What is considered simultaneous in one frame of reference will not necessarily be considered simultaneous in another. To implement a relationist theory of simultaneity in the framework of Minkowski spacetime would require that whatever laws apply to the spatial relations for one particular decomposition of spacetime must also apply to all other decompositions (Brown, 2018). That is, one would have to determine which events were simultaneous to determine the relations. But this is evidently not the case in Einstein’s solution of Lorentz Invariance which states that ‘frames in relative motion can agree on the velocity of light only if they disagree on simultaneity; only the relativity of simultaneity makes possible the invariance [i.e. ‘identicality’] of the velocity of light’ (DiSalle, 2018). Therefore, STR only works if we revoke a relationist theory of simultaneity.

4. Objections and replies

The relationist may object to my first observation by denying the independence or fundamentality of space-time structure. The relationist may do this by positing only ‘particles and the special relativistic spatiotemporal relations that exist between the events on their world-lines’ that are irreducible brute facts of nature (Maudlin, 1993, p196). At first glance, this is a fair objection given that absolutists cannot object to it by virtue of its appeal to brute facts of nature given that a Minkowskian absolutist holds that the geometry of spacetime itself is a brute fact of nature.

However, this objection does not vindicate the sort of relationism that Leibniz sought since not all motion is not considered ‘relative to some body’. There are well-defined absolute accelerations and rotations (Huggett and Hoefer, 2018). Further, in the context of STR, this objection is not persuasive. This is because it is the absoluteness of Newtonian space and of Minkowski spacetime that allows the relationist to treat them as ‘felicitous falsehoods’ whose mathematical utility is worth exploiting (p199; Elgin, 2004, p129). It is the geometrical structure of both spacetimes, which is fixed independently of the matter they comprise, that relationists can investigate a priori the nature of the manifold into which the particle trajectories and relations are embedded (Maudlin, 1993, p199).

5. Conclusion

To conclude, I have argued that while STR replaced Newton’s notion of absolute space, fixed independently of the bodies it comprises, and absolute rest, it conceptualises spacetime as being an entity in its own right. This was evidenced in the Twins Paradox and Twin Globes experiment. Even though we can describe both phenomena without reference to a privileged frame, the geometry of spacetime carries the explanatory power that a strictly relationist theory lacks. It seems that if we accept STR, we must adopt the metaphysical position advocated by Newton (i.e. substantivalism) but with Newton’s absolute space and time replaced by a substantival Minkowski spacetime. Like Maudlin (2012), I contend that despite space-time structure not being directly observable, it nonetheless plays an essential role in the formulation of physical theory (p66).

While I am wary of the risks of leaping from an unobservable postulate (some might call it a metaphysical postulate) to an empirical theory through an inference to the best explanation (Lipton, 1990, p53), I recognise that physicists rely on unobservable postulates to explain observable phenomena (Maudlin, 2012, p46). An anti-realist stance would save my argument from foreclosing the empirical possibility that STR only partially captures facts about reality (Curd, 2013, p872).

Word count: 3467

References

1. Arthur, R. T. (2010). Minkowski’s proper time and the status of the clock hypothesis. In Space, time, and spacetime. Springer, Berlin, Heidelberg. Available at: https://www.humanities.mcmaster.ca/~rarthur/papers/Mptatch.pdf [Accessed 16 Mar. 2019]
2. Brown, K. (2018). Immovable Spacetime. [online] Mathpages.com. Available at: https://www.mathpages.com/rr/s4-01/4-01.htm [Accessed 23 Mar. 2019].
3. Curd, M., & Psillos, S. (Eds.). (2013). The Routledge companion to philosophy of science. Routledge.
4. Dalarsson, M., & Dalarsson, N. (2015). Tensors, relativity, and cosmology. Academic Press.
5. Dasgupta, S. (2015). Substantivalism vs relationalism about space in classical physics. Philosophy Compass, 10(9), 601–624.
6. DiSalle, R. (2018). Space and Time: Inertial Frames”. [online] The Stanford Encyclopedia of Philosophy. Available at: https://plato.stanford.edu/cgi-bin/encyclopedia/archinfo.cgi?entry=spacetime-iframes&archive=spr2018 [Accessed 16 Mar. 2019].
7. Elgin, C. Z. (2004). True enough. Philosophical issues, 14, 113–131.
8. Gardner, M. R. (1977). Relationism and Relativity. The British Journal for the Philosophy of Science, 28(3), 215–233.
9. Huggett, N. and Hoefer, C. (2018). Absolute and Relational Theories of Space and Motion. [online] The Stanford Encyclopedia of Philosophy. Available at: https://plato.stanford.edu/entries/spacetime-theories/#9 [Accessed 23 Mar. 2019].
10. Lipton, P. (1990). Prediction and prejudice. International Studies in the Philosophy of Science, 4(1), 51–65.
11. Maudlin, T. (2012). Philosophy of physics: Space and time(Vol. 5). Princeton University Press.
12. Newton, I. (1999). The Principia: mathematical principles of natural philosophy. Univ of California Press.
13. Pooley, O. (2001). Relationism rehabilitated? ii: Relativity.
14. Sklar, L. (1974). Space, time, and spacetime. Berkeley and Los Angeles.
15. Teller, P. (1991). Substance, relations, and arguments about the nature of space-time. The Philosophical Review, 100(3), 363–397.